Vertex and Roots of Parabola

Vertex and Roots of Parabola

A parabola can cross the x-axis once, twice, or never. These points of intersection are called x-intercepts or zeros. You can find these points by putting y =0.

Now vertex of the parabola may or may not lie on the x-axis. In the case when the vertex of the parabola does not lie on the x-axis then we need to find the x-intercepts.

For example:

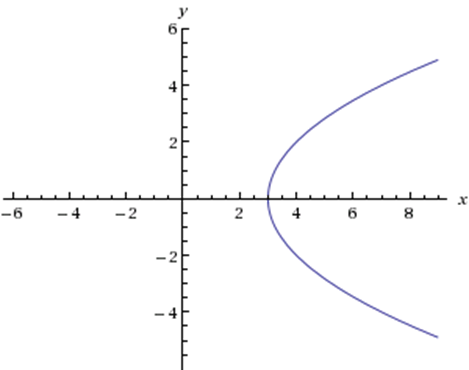
vertex of the graph lies on the x-axis
1

In this graph, the vertex of the graph lies on the x-axis.

y2=4(x-3)

Now the vertex of this parabola is (3, 0), and when u put y =0 to find the x-intercept it is again x= 3

  •  
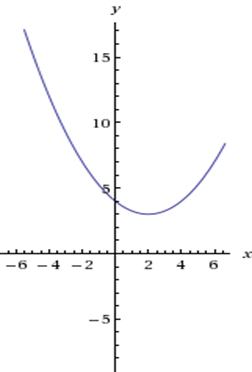
In this graph, the vertex of the graph is at (2, 3) but when we put y=0 to find the roots of the parabola, there are no real roots. You can see that this parabola do not touch the x-axis.
2

In this graph, the vertex of the graph is at (2, 3) but when we put y=0 to find the roots of the parabola, there are no real roots. You can see that this parabola do not touch the x-axis.

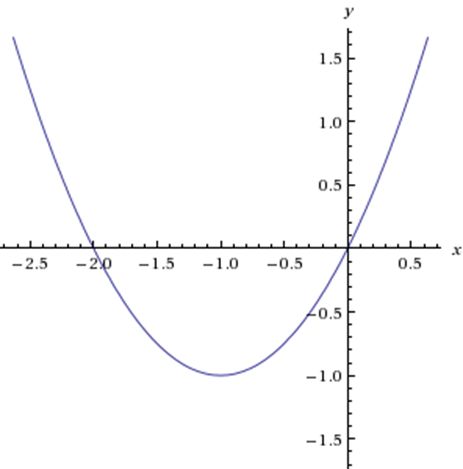
In this graph vertex of the parabola is at (-1, -1) but when we put y=0 to find the roots of the parabola, there are two real roots which are 0 and -2.
3

In this graph vertex of the parabola is at (-1, -1) but when we put y=0 to find the roots of the parabola, there are two real roots which are 0 and -2.

Related Articles: